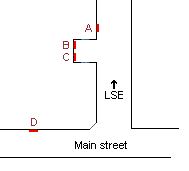
There is a bank on campus at LSE. It has four cashpoints (as the Poms call them, or ATMs to the rest of us), arranged like this:
There is frequently a queue to use the cashpoints at A and D, but almost never at B or C. They are, at most, four metres from cashpoint A, but at all times they either have no queue, or if they do, it is always shorter than that for A or D (since they are next to each other, they typically produce a single queue). This includes those times when it is raining, despite the fact that B and C are under cover, while A and D are exposed.
This poses a puzzle. Why do B and C not get used more? Why are the queues at A and D longer than they need to be?
Part of the answer lies in this next bit of information:
B and C are readily visible from the street if you stand in front of the entrance, but they are not immediately visible from a little way along the street. In particular, they are not visible from the queues that build up for cashpoints A and D. Cashpoint A is not immediately obvious when looking up from the main street.
The standard economic answer would therefore involve search costs and cut-off thresholds. There is a time (and annoyance) cost involved in checking other cashpoints and there is no guarantee that you will find one with a shorter queue. Provided that the time cost of staying with your current queue is below your reservation cost (the threshold), it’s optimal for you to stay where you are.
Most people that use D are passers-by that just happened to be walking along the main street and won’t be aware that A, B or C exist. For these people, the believed search costs could be quite high (there is not another bank in the immediate area) and the prior belief on the probability of finding a cashpoint with a shorter queue quite low (since people generally want to use cashpoints at the same time).
But the people that use A, B and C are generally all LSE staff and students who are well aware of all four cashpoints. For those waiting at A, the search cost for checking B and C is vanishingly small and for the sufficiently observant of them, their prior beliefs on the queue length at B and C will be that they are quite likely to be shorter.
So why don’t they do it?
Economists aren’t rational 🙂